여러 곳에서 자료를 모으는 중입니다.
현재 아래 자료는 페르마의 마지막 정리를 증명한 앤드류 와일즈 교수님의 강의록을 발췌한 것입니다.
#1
Fermat equation:
xn + yn = zn.
Problem: to show that there are no solutions x, y, z ∈ ℤ with
xyz ≠ 0 if n ≥ 3.
#2. Fermat's results
n = 3:
equivalent to showing there is no right-anged triangle with rational length sides and area 1
claimed in letters to Digby and Carcavi
n = 4:
no solution to x4 + y4 = z2
proved by Fermat
Fermat uses method of infinite descent (무한강하법).
무한강하법은 예를 들면 루트 2가 무리수인 것을 증명할 때 사용됨
#3. Kummer (1840's)
zp = xp + yp = (x + ζ0 y) × ··· × (x + ζp-1 y), ζ = exp(2πi / p)
Idea: use (x + ζy)(1 + ζ) - (x + ζ2 y) = ζ(x + y)
Need arithmetic of ℤ[ζ]:
(i) units;
(ii) ideal class groups.
Ideals: M·ℤ[ζ] ⊂ Ω ⊂ ℤ[ζ] (some M ∈ ℤ) such that αΩ ⊂ Ω if α ∈ ℤ[ζ].
Example: principal ideals Ω = β·ℤ[ζ].
Ideal class group: Cℓℤ[ζ] = Ideals / Principal ideals
Kummer proved
(i) 페르마의 마지막 정리는 참 if p ∤ #Cℓℤ[ζ]
(ii) p | #Cℓℤ[ζ] ⇔ p | B2 × ··· × Bp-3 (단, Bi는 베르누이 계수)
where t / (exp(t) - 1) = 1 + B0 t0 / 0! + ··· + Bn tn / n! + ···
Mazur-W: fine structure of Cℓℤ[ζ] is described by Bi's
#4.Abelian extensions
ζp satisfies (xp - 1) / (x - 1) = xp-1 + ... + 1 = 0
Gal(ℚ(ζp)/ℚ) ⭇ (ℤ / pℤ)x
{σℓ : ζp → ζpℓ} ↔ ℓ mod p
The Galois group is abelian.
Call σℓ the Frobenius at ℓ (Frobℓ).
Theoren (Kronecker-Weber)
Get all abelian extensions of ℚ from roots of 1.
#5. Class field theory (Hilbert, Takagi, Artin, Hasse, ...)
Describes abelian extensions of anu number field.
Example: F = ℚ(21/3)
∃ Fp such that
Gal(Fp / F) ≃ (OF / pOF)x / (image of OF,+x)
where OF = ℤ [21/3], and OF,+x = units of OF
More complicated when ideal class group of OF is not 1.
#6. Non-abelian approaches
Frey (1985): New idea! Suppose ap + bp = cp, p prime ≥ 3, a, b, c ∈ ℤ
Consider y2 = x(x - ap)(x + bp).
Discriminant is (apbpcp)2.
Such a curve should not exist!
Discriminants should not be pth powers.
New way to prove F.L.T. (페르마의 마지막 정리): show there is no such elliptic curve.
#7. Elliptic curves
E : y2 = x3 + Ax + B (A, B ∈ ℚ)
E(ℂ) ≃ ℂ / Λ is a group, Λ = ℤ + ℤ.τ
E(ℂ)[pn] ≃ ℤ/p^nℤ⊕ℤ/pnℤ
Gal(ℚ(E[pn])/ℚ) ↪ GL2(ℤ/pnℤ)
Algebraically: these are the 8 points of inflection of
y2 = x3 + Ax + B plut the 9th one at ∞.
The coordinates generate a field ℚ(E[3])
Gal(ℚ(E[3])/ℚ) ↪ GL2(ℤ/3ℤ).
#8. Modular forms
Modur forms: functions
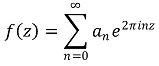
satisfying
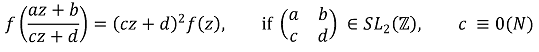
Theorem (Modularity conjecture of Taniyama & Shimura)

#9. Rough over view of proof
어려운 페르마의 마지막 정리를 풀기 위해 더 어려운 타원곡선 문제를 증명
(Frey &) Ribet: Modularity conjecture → F. L. T
Problem : prove modularity conjecture
First step : consider
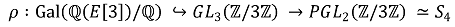
Langlands (& Tunnell) : this is connected with a modular form. Based on his theory of cyclic base change: note S4 is a solvable group.
#10. Langlands' programme
Generalisation of class field theory:
Describe all Galois extensions of ℚ (or any number field).
Generalises modular forms to automorphic representations:
roughly speaking we use functions invariant under subgroups of GLn(OF) to classify n-dimensional representations of
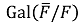
(Better to use adeles and adelic representation theory).
#11. A little progress...
Theorem (Khare-Wintenberger)
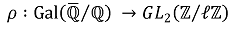
Assume det ρ is odd, ρ irreducible.
Then ρ is associated to a modular form.
Proof uses similar techniques with an ingenious induction on ℓ.
Does not seem to work in the general case (GLn and any F in place of ℚ).
'▶ Drafts' 카테고리의 다른 글
| 여분 (0) | 2023.03.12 |
|---|---|
| 여분 (0) | 2018.03.25 |
| 여분 (0) | 2016.06.25 |
| 【수학】 수학 메모 (0) | 2016.06.24 |
| 【상식】 상식 노트 (0) | 2016.06.23 |

최근댓글