The Solution for GRE Mathematics Practice Test [01-20]
추천글 : 【GRE】 Solution for GRE Mathematics Practice Test
1.
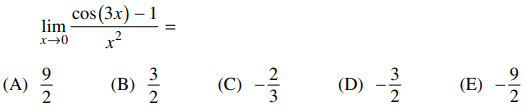
⑴ answer: E
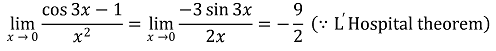
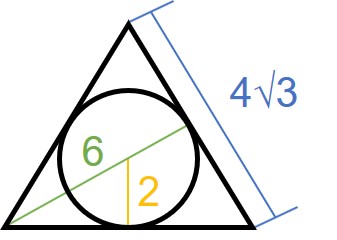
2. What is the area of an equilateral triangle whose inscribed circle has radius 2?
⑴ answer: C
3.
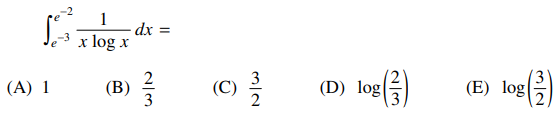
⑴ answer: D
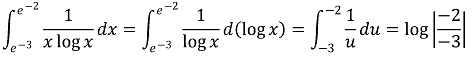
4. Let V and W be 4-dimensional subspaces of a 7-dimensional vector space X. Which of the following CANNOT be the dimension of the subspace V ∩ W?

⑴ answer: A
⑵ V = span [{e1, e2, e3, e4}], W = span [{e1, e2, e3, e4}] → dim(V ∩ W) = 4
⑶ V = span [{e1, e2, e3, e4}], W = span [{e1, e2, e3, e7}] → dim(V ∩ W) = 3
⑷ V = span [{e1, e2, e3, e4}], W = span [{e1, e2, e6, e7}] → dim(V ∩ W) = 2
⑸ V = span [{e1, e2, e3, e4}], W = span [{e1, e5, e6, e7}] → dim(V ∩ W) = 1
5. Sofia and Tess will each randomly choose one of the 10 integers from 1 to 10. What is the probability that neither integer chosen will be the square of the other?
⑴ answer: E
⑵ the number of cases that either integer chosen will be the square of the other : (1, 1), (2, 4), (3, 9), (4, 2), (9, 3)
⑶ the probability : 1 - (5 / 100) = 0.95
6. Which of the following shows the numbers 21/2, 31/3, and 61/6 in increasing order?
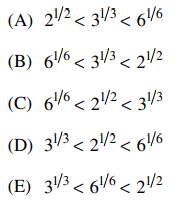
⑴ answer: C
⑵ 6 < 23 = 8 ⇔ 61/6 < 21/2
⑶ 23 = 8 < 32 = 9 ⇔ 21/2 < 31/3
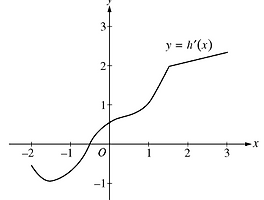
7. The figure below shows the graph of the derivative f ' of a function f, where f is continuous on the interval [0, 4] and differentiable on the interval (0, 4). Which of the following gives the correct ordering of the values f (0), f (2), and f (4)?
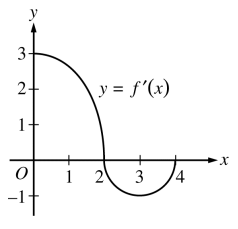
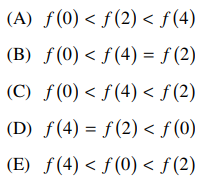
⑴ answer: C
⑵ f (0) = f (2) - ∫ f ' dx (x = 0 to 2) < f (2) + ∫ f ' dx (x = 2 to 4) = f (4)
⑶ f (2) + ∫ f ' dx (x = 2 to 4) = f (4) < f (2)
8. Which of the following is NOT a group?

⑴ answer: B
⑶ In case of (B), no inverse element always exists, so it is not a group.
9. Let g be a continuous real-valued function defined on ℝ with the following properties.
g '(0) = 0
g ''(-1) > 0
g ''(x) < 0 if 0 < x < 2.
Which of the following could be part of the graph of g?

⑴ answer: A
⑵ A : g '(0) = 0, g ''(-1) > 0 (convex), g ''(x) < 0 if 0 < x < 2
⑶ B : g '(0) = 0, g ''(-1) > 0 (convex), a point of inflection exists at 0 < x < 2
⑷ C : g '(0) > 0, g ''(-1) > 0 (convex), g ''(x) < 0 if 0 < x < 2
⑸ D : g '(0) = 0, g ''(-1) > 0 (convex), a point of inflection exists at 0 < x < 2
⑹ E : g '(0) = 0, g ''(-1) < 0 (concave), g ''(x) < 0 if 0 < x < 2
10. In the xy-plane, the set of points whose coordinates satisfy the equation below is
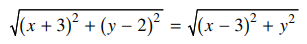

⑴ answer: A
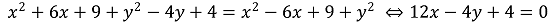
11. The region bounded by the curves y = x and y = x2 in the first quadrant of the xy-plane is rotated about the y-axis. The volume of the resulting solid of revolution is
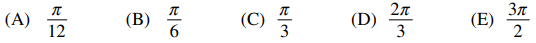
⑴ answer: B
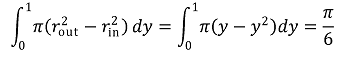
12. For which integers n such that 3 ≤ n ≤ 11 is there only one group of order n (up to isomorphism)?

⑴ answer: B
⑵ There is only one group of order n if gcd(n, φ(n))=1, φ being Eular's totient function.
○ φ(3) = 3 × (1 - 1/3) = 2
○ φ(4) = 4 × (1 - 1/2) = 2
○ φ(5) = 5 × (1 - 1/5) = 4
○ φ(6) = 6 × (1 - 1/2) × (1 - 1/3) = 2
○ φ(7) = 7 × (1 - 1/7) = 6
○ φ(8) = 8 × (1 - 1/2) = 4
○ φ(9) = 9 × (1 - 1/3) = 6
○ φ(10) = 10 × (1 - 1/2) × (1 - 1/5) = 4
○ φ(11) = 11 × (1 - 1/11) = 10
13. If f is a continuously differentiable real-valued function defined on the open interval (-1, 4) such that f (3) = 5 and f '(x) ≥ -1 for all x, what is the greatest possible value of f (0)?
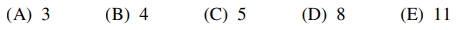
⑴ answer: D
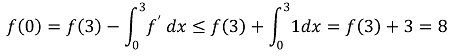
14. Suppose g is a continuous real-valued function such that
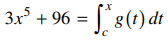
for each x ∈ ℝ, where c is a constant. What is the value of c?
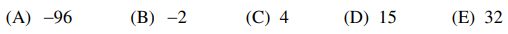
⑴ answer: B
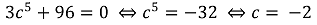
15. Let S, T, and U be nonempty sets, and let f : S → T and g : T → U be functions such that the function g ∘ f : S → U is one-to-one (injective). Which of the following must be true?
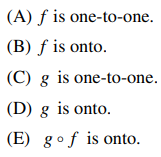
⑴ answer: A
⑵ if f is not one-to-one, g(f(x)) = g(f(y)) even if x ≠ y.
16. Suppose A, B, and C are statements such that C is true if exactly one of A and B is true. If C is false, which of the following statements must be true?

⑴ answer: B
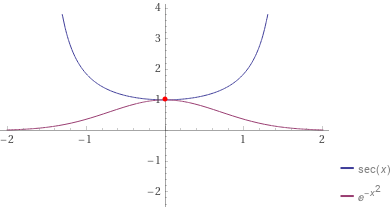
17. Which of the following equations has the greatest number of real solutions?
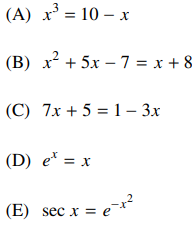
⑴ answer: B
⑵ A : one solution. The graph shows that there is one solution.
⑶ B : two solution. The root formula.
⑷ C : one solution
⑸ D : no solution. For x < 0, ex > 0 > x. For x > 0, (ex)' = ex > e0 = 1.
⑹ E : one solution at x = 0
18. Let f be the function defined by
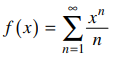
for all x such that -1 < x < 1. Then f '(x) =
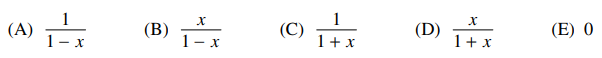
⑴ answer: A
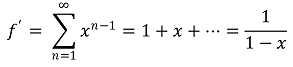
19. If z is a complex variable and z̄ denotes the complex conjugate of z, what is
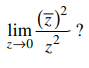
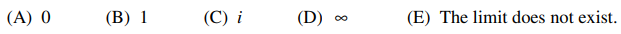
⑴ answer: E
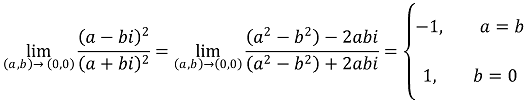
20. Let g be the function defined by g(x) = e2x+1 for all real x. Then
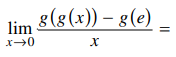
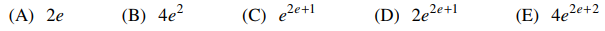
⑴ answer: E
⑵ the definition of derivation
입력: 2023.01.14 16:34
'▶ 자연과학' 카테고리의 다른 글
| 【GR1768】 The Solution for GRE Mathematics Practice Test [41-60] (0) | 2023.01.21 |
|---|---|
| 【GR1768】 The Solution for GRE Mathematics Practice Test [21-40] (0) | 2023.01.21 |
| 【GR1776】 The Solution for GRE Physics Practice Test [81-100] (0) | 2023.01.01 |
| 【GR1776】 The Solution for GRE Physics Practice Test [61-80] (0) | 2022.12.30 |
| 【GR1776】 The Solution for GRE Physics Practice Test [41-60] (0) | 2022.12.28 |


최근댓글