【국제수학올림피아드】 IMO 기하 문제 풀이 (2020년 ~ 2024년)

IMO 기하 문제 풀이 (2020년 ~ 2024년)

 

추천글 : 【기하학】 IMO 기하 문제 풀이 종합 

---

 

IMO 2020, Problem 1. Consider the convex quadrilateral ABCD. The point P is in the interior of ABCD. The following ratio equalities hold:

 

∠PAD : ∠PBA : ∠DPA = 1 : 2 : 3 = ∠CBP : ∠BAP : ∠BPC.

 

Prove that the following three lines meet in a point: the internal bisectors of angles ∠ADP and ∠PCB and the perpendicular bisector of segment AB.

 

○ 풀이. 추후 업데이트

 

 

IMO 2021, Problem 3. Let D be an interior point of the acute triangle ABC with AB > AC so that ∠DAB = ∠CAD. The point E on the segment AC satisfies ∠ADE = ∠BCD, the point F on the segment AB satisfies ∠FDA = ∠DBC, and the point X on the line AC satisfies CX = BX. Let O₁ and O₂ be the circumcenters of the triangles ADC and EXD, respectively. Prove that the lines BC, EF, and O₁O₂ are concurrent.

 

○ 풀이. 추후 업데이트

 

 

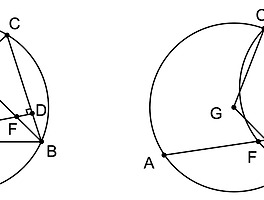
IMO 2021, Problem 4. Let Γ be a circle with center I, and ABCD a convex quadrilateral such that each of the segments AB, BC, CD and DA is tangent to Γ. Let Ω be the circumcircle of the triangle AIC. The extension of BA beyond A meets Ω at X, and the extension of BC beyond C meets Ω at Z. The extensions of AD and CD beyond D meet Ω at Y and T, respectively. Prove that

 

AD + DT + TX + XA = CD + DY + YZ + ZC.

 

○ 풀이. 추후 업데이트

 

 

IMO 2022, Problem 4. Let ABCDE be a convex pentagon such that BC = DE. Assume that there is a point T inside ABCDE with TB = TD, TC = TE and ∠ABT = ∠TEA. Let line AB intersect lines CD and CT at points P and Q, respectively. Assume that the points P, B, A, Q occur on their line in that order. Let line AE intersect CD and DT at points R and S, respectively. Assume that the points R, E, A, S occur on their line in that order. Prove that the points P, S, Q, R lie on a circle.

 

○ 풀이. AlphaGeometry (DDAR mode)

 

==========================
 * From theorem premises:
B C D E T A P Q R S : Points
ED = BC [00]
TC = TE [01]
TB = TD [02]
∠TBA = ∠AET [03]
P,A,B are collinear [04]
D,P,C are collinear [05]
Q,A,B are collinear [06]
Q,T,C are collinear [07]
E,A,R are collinear [08]
D,R,C are collinear [09]
E,A,S are collinear [10]
D,T,S are collinear [11]

 * Auxiliary Constructions:
: Points


 * Proof steps:
001. ED = BC [00] & TC = TE [01] & TB = TD [02] (SSS)⇒  ∠EDT = ∠CBT [12]
002. ED = BC [00] & TC = TE [01] & TB = TD [02] (SSS)⇒  ∠DET = ∠BCT [13]
003. ED = BC [00] & TC = TE [01] & TB = TD [02] (SSS)⇒  ∠DTE = ∠BTC [14]
004. ∠EDT = ∠CBT [12] & D,T,S are collinear [11] ⇒  ∠EDS = ∠CBT [15]
005. ∠DET = ∠BCT [13] & Q,T,C are collinear [07] ⇒  ∠DET = ∠BCQ [16]
006. E,A,S are collinear [10] & Q,A,B are collinear [06] & ∠AET = ∠TBA [03] ⇒  ∠SET = ∠TBQ [17]
007. D,T,S are collinear [11] & Q,T,C are collinear [07] & ∠DTE = ∠BTC [14] ⇒  ∠STE = ∠BTQ [18]
008. ∠SET = ∠TBQ [17] & ∠STE = ∠BTQ [18] (Similar Triangles)⇒  TS:TQ = TE:TB [19]
009. TS:TQ = TE:TB [19] & TB = TD [02] & TC = ET [01] ⇒  TS:TQ = TC:TD [20]
010. D,T,S are collinear [11] & Q,T,C are collinear [07] ⇒  ∠STQ = ∠DTC [21]
011. TS:TQ = TC:TD [20] & ∠STQ = ∠DTC [21] (Similar Triangles)⇒  ∠TSQ = ∠DCT [22]
012. ∠TSQ = ∠DCT [22] & D,T,S are collinear [11] & Q,T,C are collinear [07] ⇒  ∠DSQ = ∠DCQ [23]
013. ∠TBA = ∠AET [03] & ∠EDS = ∠CBT [15] & ∠DET = ∠BCQ [16] & ∠DSQ = ∠DCQ [23] (Angle chase)⇒  ∠(AE-CD) = ∠(QS-AB) [24]
014. Q,A,B are collinear [06] & P,A,B are collinear [04] & E,A,S are collinear [10] & E,A,R are collinear [08] & D,R,C are collinear [09] & D,P,C are collinear [05] & ∠(QS-AB) = ∠(AE-CD) [24] ⇒  ∠SQP = ∠SRP [25]
015. ∠SQP = ∠SRP [25] ⇒  Q,S,P,R are concyclic
==========================

 

○ 풀이. AlphaGeometry (AlphaGeometry mode)

 

==========================
 * From theorem premises:
A B C D E F G H I J : Points
DC = AB [00]
EA = EC [01]
EB = ED [02]
∠EAF = ∠FDE [03]
C,G,B are collinear [04]
F,G,A are collinear [05]
F,H,A are collinear [06]
H,B,E are collinear [07]
C,I,B are collinear [08]
F,I,D are collinear [09]
F,J,D are collinear [10]
C,J,E are collinear [11]

 * Auxiliary Constructions:
: Points


 * Proof steps:
001. DC = AB [00] & EA = EC [01] & EB = ED [02] (SSS)⇒  ∠ABE = ∠CDE [12]
002. DC = AB [00] & EA = EC [01] & EB = ED [02] (SSS)⇒  ∠BAE = ∠DCE [13]
003. DC = AB [00] & EA = EC [01] & EB = ED [02] (SSS)⇒  ∠AEB = ∠CED [14]
004. F,J,D are collinear [10] & F,H,A are collinear [06] & ∠FDE = ∠EAF [03] ⇒  ∠JDE = ∠EAH [15]
005. C,J,E are collinear [11] & H,B,E are collinear [07] & ∠CED = ∠AEB [14] ⇒  ∠JED = ∠AEH [16]
006. ∠JDE = ∠EAH [15] & ∠JED = ∠AEH [16] (Similar Triangles)⇒  EJ:EH = ED:EA [17]
007. EJ:EH = ED:EA [17] & EA = EC [01] & BE = DE [02] ⇒  EB:EC = EJ:EH [18]
008. H,B,E are collinear [07] & C,J,E are collinear [11] ⇒  ∠BEC = ∠HEJ [19]
009. EB:EC = EJ:EH [18] & ∠BEC = ∠HEJ [19] (Similar Triangles)⇒  ∠EBC = ∠HJE [20]
010. ∠EBC = ∠HJE [20] & C,J,E are collinear [11] ⇒  ∠EBC = ∠(HJ-CE) [21]
011. ∠EAF = ∠FDE [03] & ∠ABE = ∠CDE [12] & ∠BAE = ∠DCE [13] & ∠EBC = ∠(HJ-CE) [21] (Angle chase)⇒  ∠(DF-HJ) = ∠(BC-AF) [22]
012. C,I,B are collinear [08] & C,G,B are collinear [04] & F,H,A are collinear [06] & F,G,A are collinear [05] & F,J,D are collinear [10] & F,I,D are collinear [09] & ∠(BC-AF) = ∠(DF-HJ) [22] ⇒  ∠IGH = ∠IJH [23]
013. ∠IGH = ∠IJH [23] ⇒  H,I,J,G are concyclic
==========================

 

입력: 2024.05.05 21:54